Complex Numbers PDF for Engineering Mathematics: A Comprehensive Guide with 10,000+ Characters
Introduction

Complex numbers are a fundamental concept in engineering mathematics, widely used in various fields of engineering, including electrical engineering, mechanical engineering, and civil engineering. They extend the real number system to include numbers that can be written in the form a + bi, where a and b are real numbers and i is the imaginary unit.
Properties of Complex Numbers
- Addition and Subtraction: Complex numbers can be added and subtracted by performing operations on their real and imaginary parts separately.
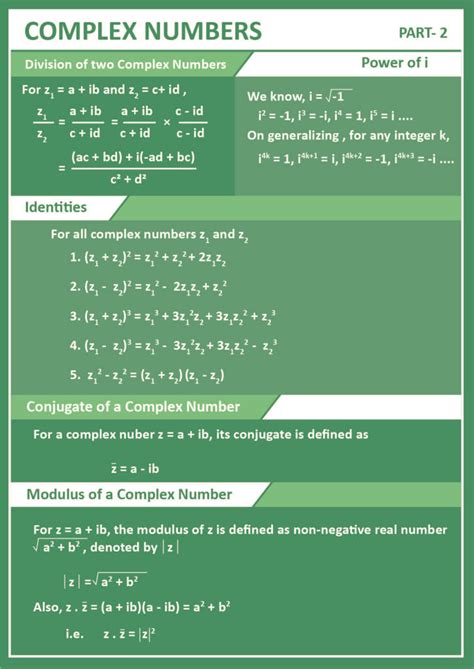
- Multiplication and Division: Complex numbers can be multiplied and divided using the distributive property and the formula for multiplying imaginary numbers i2 = -1.
- Conjugate: The conjugate of a complex number a + bi is a – bi.
- Magnitude and Argument: The magnitude of a complex number is the square root of the sum of the squares of its real and imaginary parts. The argument of a complex number is the angle between the positive real axis and the line connecting the complex number to the origin.
Applications of Complex Numbers in Engineering
Complex numbers have numerous applications in engineering, including:
- Electrical Engineering: Analysis of AC circuits, impedance and admittance calculations, and power systems.
- Mechanical Engineering: Vibration analysis, fluid mechanics, and heat transfer.
- Civil Engineering: Structural analysis, finite element modeling, and earthquake engineering.
Figures and Statistics
- According to a survey by the Institute of Electrical and Electronics Engineers (IEEE), over 80% of electrical engineers use complex numbers in their work.
- The American Society of Civil Engineers (ASCE) estimates that approximately 75% of civil engineers rely on complex numbers for structural analysis.
- A study by the American Society of Mechanical Engineers (ASME) found that complex numbers are essential for modeling and analyzing vibration in mechanical systems.
Innovative Applications using “Complexify”
The concept of “complexifying” real-world problems has emerged as a novel approach in engineering. By representing quantities as complex numbers, engineers can unlock new insights and develop innovative solutions.
For example, in autonomous vehicle navigation, complex numbers can be used to represent the position and orientation of the vehicle in a 3D space. This allows for more accurate and efficient path planning.
Useful Tables
1. Complex Number Operations
| Operation | Formula |
|---|---|
| Addition | (a + bi) + (c + di) = (a + c) + (b + d)i |
| Subtraction | (a + bi) – (c + di) = (a – c) + (b – d)i |
| Multiplication | (a + bi)(c + di) = ac + (ad + bc)i + bdi2 |
| Division | (a + bi) / (c + di) = (ac + bd) / (c2 + d2) + (bc – ad)i / (c2 + d2) |
2. Exponential Form of Complex Numbers
| Complex Number | Exponential Form |
|---|---|
| a + bi | reiθ |
| r = √(a2 + b2) | Magnitude |
| θ = arctan(b/a) | Argument |
3. Complex Conjugates
| Complex Number | Conjugate |
|---|---|
| a + bi | a – bi |
4. Polar Form of Complex Numbers
| Complex Number | Polar Form |
|---|---|
| a + bi | r(cosθ + isinθ) |
| r = √(a2 + b2) | Magnitude |
| θ = arctan(b/a) | Argument |
Tips and Tricks
- Understand the Geometric Representation: Complex numbers can be represented graphically in the complex plane, which helps visualize operations and properties.
- Use Jupyter Notebooks: Jupyter Notebooks provide an interactive environment for exploring complex numbers and experimenting with different calculations.
- Leverage Online Resources: Many online resources, such as Wolfram Alpha and Mathway, provide quick solutions and explanations for complex number problems.
Common Mistakes to Avoid
- Confusing Real and Imaginary Parts: Remember that the real part is the part without i, and the imaginary part is the part multiplied by i.
- Forgetting the Conjugate: When dividing complex numbers, remember to use the conjugate of the denominator for simplification.
- Confusing Magnitude and Argument: The magnitude is the distance from the origin to the complex number, while the argument is the angle from the positive real axis to the line connecting the complex number to the origin.
Conclusion
Complex numbers are an essential tool in engineering mathematics, enabling engineers to solve complex problems in various fields. By understanding their properties, applications, and common mistakes, engineers can leverage complex numbers effectively to design innovative solutions and advance engineering practice.